Two Examples of Gage Run Chartmain topic interpreting results session command see also
Two Examples of Gage Run ChartIn this example, you draw a gage run chart with two data sets: one in which measurement system variation contributes little to the overall observed variation (GAGEAIAG.MTW), and one in which measurement system variation contributes a lot to the overall observed variation (GAGE2.MTW). For comparison, see the same data sets analyzed by the Gage R&R Study - ANOVA Method or the Gage R&R Study - Xbar and R method.
The GAGEAIAG data was taken from Measurement Systems Analysis Reference Manual, 3rd edition. (Chrysler, Ford, General Motors Supplier Quality Requirements Task Force). Ten parts were selected that represent the expected range of the process variation. Three operators measured the ten parts, three times per part, in a random order.
For the GAGE2 data, three parts were selected that represent the expected range of the process variation. Three operators measured the three parts, three times per part, in a random order.
Step 1: Gage Run Chart with GAGEAIAG data
1 Open the worksheet GAGEAIAG.MTW.
2 Choose Stat > Quality Tools > Gage Study > Gage Run Chart.
3 In Part numbers, enter Part.
4 In Operators, enter Operator.
5 In Measurement data, enter Measurement. Click OK.
Step 2: Gage Run Chart with GAGE2 data
1 Open the file GAGE2.MTW.
2 Choose Stat > Quality Tools > Gage Study > Gage Run Chart.
3 In Part numbers, enter Part.
4 In Operators, enter Operator.
5 In Measurement data, enter Response. Click OK.
Graph window output
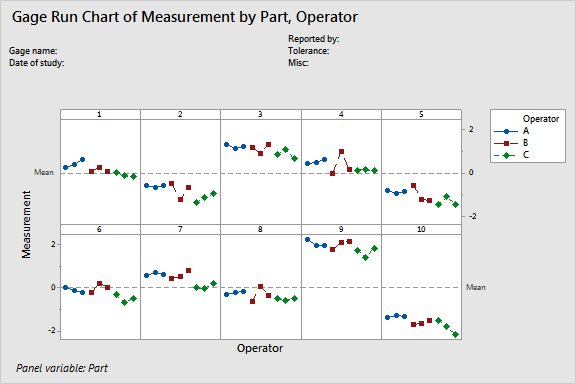
Gage Run Chart with GAGEAIAG data
Gage Run Chart with GAGE2 data
For each part, you can compare both the variation between measurements made by each operator, and differences in measurements between operators.
You can also look at the measurements in relationship to the horizontal reference line. By default the reference line is the mean of all observations.
Most of the variation is due to differences between parts. Some smaller patterns also appear. For example, Operator B does not measure consistently and Operator C usually measures lower than the other operators.
For each part, you can compare both the variation between measurements made by each operator, and differences in measurements between operators.
You can also look at the measurements in relationship to the horizontal reference line. By default the reference line is the mean of all observations.
The dominant factor here is repeatability - large differences in measurements when the same operator measures the same part. Oscillations might suggest the operators are "adjusting" how they measure between measurements.