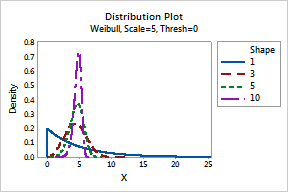
Effect of the shape parameter
Effect of the scale parameter

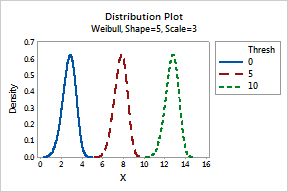
Effect of the threshold parameter
Commonly used to model time-to-failure data. For example, a manufacturer is interested in calculating the probability that a part fails after one, two, or more years. It has wide application in engineering, medical research, finance, and climatology.
Weibull distribution is described by the shape, scale, and threshold parameters. The case when the threshold parameter is zero is called the 2-parameter Weibull distribution. It is defined only for nonnegative variables.
Depending on the values of the parameters, the Weibull distribution can take various shapes.
|
Effect of the shape parameter |
Effect of the scale parameter |
|
|
|
|
Effect of the threshold parameter |
|
|
|
|
One of the main advantages of this distribution is that it can take on the characteristics of other types of distribution making it extremely flexible in fitting different kinds of data. For example,
When the shape parameter is 2, the Rayleigh distribution can be derived from the Weibull distribution.